Staff across National Museums Scotland are sharing how important maths is in their work as part of Maths Week Scotland's #ShowYourWorking campaign. Why not have a go at some of the activities below! Let us know how you get on @NMSEngage and @MathsWeekScot on Twitter.
Dan Potter - Assistant Curator for Ancient Medieval Collections
Dan is the Assistant Curator for Ancient Mediterranean collections. He helps to look after, share & research objects from the ancient world. His specialism is Egyptology, which includes reading hieroglyphs!
These activities are inspired by Dan's work here at the National Museum of Scotland. As part of his job he researches the history of objects, some of which were bought & sold in the antiquities market over a hundred years ago. Many different currencies were used, so it can be helpful to work out conversion rates & calculate their modern equivalents with inflation.
Conversion rates: Decimalise the British Pound (e.g. 100p in £1). If £1 GBP was worth 20 French Francs (Fr), 1 ÷ 20 tells us how much 1 Franc was worth = £0.05. This can be multiplied with the Franc rate. To calculate the value of 355 Fr do the following 0.05 x 335 = £16.75
For inflation, we aim for an annual 2% increase, but this didn’t always happen. As the rate changes every year, calculations can be very complex. Helpfully, the Bank of England have a calculator to help: http://ow.ly/OPoy50FKdaM Sometimes asking the experts is the best method!
Financial transactions that happened in the early 20th century can be hard to appreciate, especially when they are in £/s/d or French Francs which aren’t used today. Working out conversion rates or modern equivalents can help me understand & share the results of my research.
Image shows a conversion challenge which reads, ‘Conversion Challenge! Using the above calculation, what would 475 Fr be in GBP?’
Lucy Bull - Learning Enabler
Steven Dey - Creator of 3D Models
3-D Printing is the creation of a three-dimensional object from a digital 3-D model or a CAD (Computer-Aided Design) model.
3-D printing can broadly refer to several methods which create a physical object using computer control. This device can be programmed to deposit workable material (for example melted plastic) in flat, thin layers that join and gradually build up then harden.
The earlier stage of creating a digital 3-D model requires maths to get the correct measurements of the original object. Many professionals use a scanner to collect information, this can also include details about the objects surface for example the texture and/or colour.
Activity:
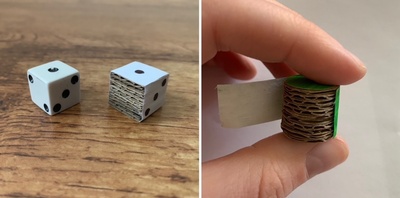
In this activity, we used mathematical tools, such as rulers and protractors, to gather information about the object we wanted to recreate as a 3-D model. We made a note of the object's length and width – for more advance models we calculated the circumference too. Once we had all the information we required, we used cardboard to replicate the 3-D printer process. To finish, we used paper and colouring pens to add a render - a visual representation of what the surface looked like. If you would like to try out this activity, follow the steps below.
What you will need:
Objects – to start off we opted for game pieces such as dice and counters, for a more advance remake we chose an egg cup. *Remember, the larger the object the longer it will take to build!
Ruler and a compass – to measure and assist when drawing
Pencil – to take note of object measurements
Paper – to record measurements and to apply a surface render
Cardboard – to build our 3-D object in layers
Scissors – to cut the cardboard and paper
Glue – to stick together the layers of cardboard and the paper surface render
Pens – to add detail to the finished surface
Step 1 (Option A)
Using the ruler, measure and take note of the object's length and width.
If the object is cylindrical, measure the diameter and half this to get the radius – you will need this information later when using the compass.
Step 1 (Option B)
If this step is too advanced, simply trace around the object and create a template.
Top Tip!
If completing a more advance build, such as an egg cup, use the compass to help you gather measurements in tricky areas.
Do this by placing the compass across the area you wish to measure and adjust the compass until it lines up with object’s edges. Then, place the compass next to the ruler to take note of the dimension it equates to.
Step 3
Draw out the shape serval times (enough to get you started) then carefully cut around the shapes.
Step 4
Stack the cardboard shapes until it matches the objects height. When ready, glue all the cardboard layers together.
Step 5
Render the surface of the cardboard. We did this by repeating Step 3 – using paper instead, then attached it to the 3-D cardboard model using glue. To finish, we used colouring pens to decorate the surface.
Hi, I’m Bob, I'm a Geologist. I work with the rock, mineral, and meteorite collection at the National Museum of Scotland. I analyse specimens in our lab to find out exactly what they are, where they are from, or how they formed.
This experiment is inspired by Bob’s #ShowYourWorking example which focuses on X-ray Diffraction.
X-rays are powerful waves of energy called radiation. Light, heat and sound are all forms of radiation; energy that can move from one place to another. But unlike x-rays, light can’t pass through objects e.g. suitcases.
For this experiment, we are going to use light as a visual representation of x-rays diffracting, or bouncing, off our mineral substitute – a mirror.
Step 1
What you will need:
Mirror – to represent a mineral sample which will ‘diffract’ (or bounce) the light rays from the torch (“x-rays”, from the “x-ray gun”) which will be detected on the “detector”.
Dark Card – to act as a “detector” to detect (or in this example, to display the unique pattern)
Torch – represents the x-ray gun in the XRD machine. The light rays will represent x-rays.
Masking tape and scissors – to create a pattern (which represents the arrangement of atoms which are unique to every mineral)
Step 2
Using masking tape and scissors, create a unique pattern. This represents the spacing of atoms which are unique to every mineral. Remember, this pattern would not be visible to the naked eye, this arrangement is of the mineral structure. X-rays can pass through this, but light rays can’t which is why we are applying it to the surface.
Step 3
To replicate the XRD machine, as seen in Bob’s video, organise the components in to a triangular arrangement.
When in use, ensure the ‘x-ray gun’ and ‘detector’ move in a symmetrical arc, keeping an equal distance.
Step 4
The white solid line represents the mineral surface.
The blue dashed line is symbolic of the x-rays leaving the x-ray gun, diffracting off the mineral surface and being detected by the detector.
The yellow solid line represents the angle used in the equation Bragg’s Law.
The red dashed line shows what would happen if the x-ray gun was in the incorrect position, therefore, the incorrect angle.
Step 5
*It is now time to conduct the experiment, dim the lights and/or close the curtains to ensure the light rays will be visible.
When ready, turn on the torch (x-ray gun) and direct it towards the mirror (mineral sample)
Ensure the triangular arrangement is kept by moving the torch (x-ray gun) and card (detector) in a symmetrical arch until the unique pattern is ‘detected’ on the card (detector).
The card only detects the beam of rays when they diffract, or bounce, across the mirror (mineral sample) at the correct angle.
*We would recommend this activity is carried out in small groups of 2+ people as angling all the components can be tricky. We used books to prop up the mirror (mineral sample) in order to photograph the result, however, it may be easier to conduct this experiment with the mirror (mineral sample) lying flat on a table. In this case, invert the triangular arrangement so the torch (x-ray gun) and card (detector) move in a symmetrical arc above.
Step 6
The unique pattern shown on the card (detector) is a representation of the light (x-rays) which were able to diffract (bounce) across the mirror (mineral sample). The result shows the unique arrangement of the mineral atoms (masking tape pattern on the mirror).
We have enjoyed seeing what everyone got up to for Maths Week Scotland this year, in particular the different ways everyone was inspired by this year's theme of 'Maths Tales'. We've picked out a few highlights to share.
For Scottish Museums Day (3 October) we’ve rounded up some of our favourite activities from museums from across the country, from this year's Maths Week Scotland.
